a.
finite energy budgets and necessary trade-offs:
1. Mutation
2. Migration
3. Non-Random
Mating:
4. Populations of Finite Size and Sampling Error
- "Genetic Drift"
We continue today with a description of why a population would deviate from Hardy-Weinberg Equilibrium. To this point, we have considered the four factors listed above. Now, we will examine the effect of selection on the genetic structure of a population.
5. Natural Selection
1. Fitness Components:
As you know, natural selection is
"differential reproductive success" in a genetically variable population.
We can measure lifetime reproductive success as the number of successfully reproductive
offspring that a genotype produces, on average. This measureable quantity is called
"fitness". There are three factors that can influence 'fitness', or
reproductive success:
Now, it seems like natural selection would favor organisms that maximized all three components; and it would if that were possible. However, organisms can't maximize all three components because there are energetic constraints - organisms only have so much energy in their 'energy budget'. So, maximizing one component results in less energy that can be invested in the other two. In addition, there are contradictory selective pressures in the environment, such that a characteristic may increase fitness with respect to one variable but decrease it with respect to another variable. These are called 'trade-offs', and we will now examine these in more detail.
2. Constraints:
a.
finite energy budgets and necessary trade-offs:
The most obvious constraint is ENERGY.
Every organism has a finite energy budget; it has harvested only so much energy
from the environment that it can allocate to all of its activities. There
are three major expenses:
So, with limited energy, increasing one thing means that you must reduce costs in another area. These patterns of energy allocation have direct effects on different fitness components.
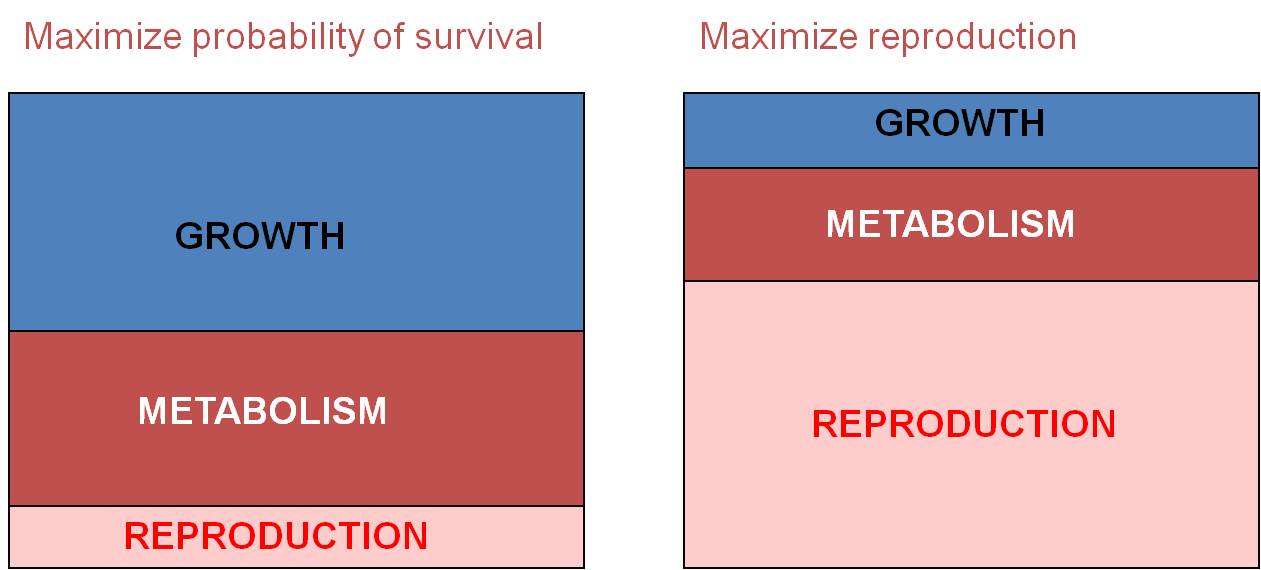Trade-Off
#1: Survival vs. Immediate Reproduction: For example, ff an organism
maximizes energy investment in growth and metabolism, then they will increase
the probability that they survive. Why? Because being large tends to increase
the probability of survival, if only because there are fewer things that can
eat you. Likewise, large organisms are not as sensitive to changes in the environment.
Simply because of their larger size (and smaller surface area/volume ratio),
they don't lose heat, salt, water, or other materials to the environment as
rapidly as small organisms. However, by investing in growth, this means that
there is LESS energy to invest in immediate reproduction, meaning fewer offspring
can be produced. So, you CAN'T maximize all three components of selection
at the same time; selection seeks the best compromise in a given environment,
with particular biological potentials and constraints. Many organisms invest
in growth when young, to develop as rapidly as possible through these early,
vulnerable life-history stages. They delay reproduction completely when
they are young to maximize growth rate. Then, after they are older and
larger, they invest energy in reproduction (and growth rate SLOWS). These
organisms are "perennial" or "K" strategists - they are long-lived
organisms. Other organisms take a different strategy. They reproduce
early at the expense of growth. They don't survive long as a consequence
- they are "annual" or "r" strategists - species that live for less
than a year but invest almost all their energy in immediate reproduction. These
two examples are opposite sides of the same coin - they are both examples of
this same trade-off between survival and immediate reproduction; organism can't
maximize both at the same time, so they tend to maximize one or the other, or
change their pattern of allocation through their life.
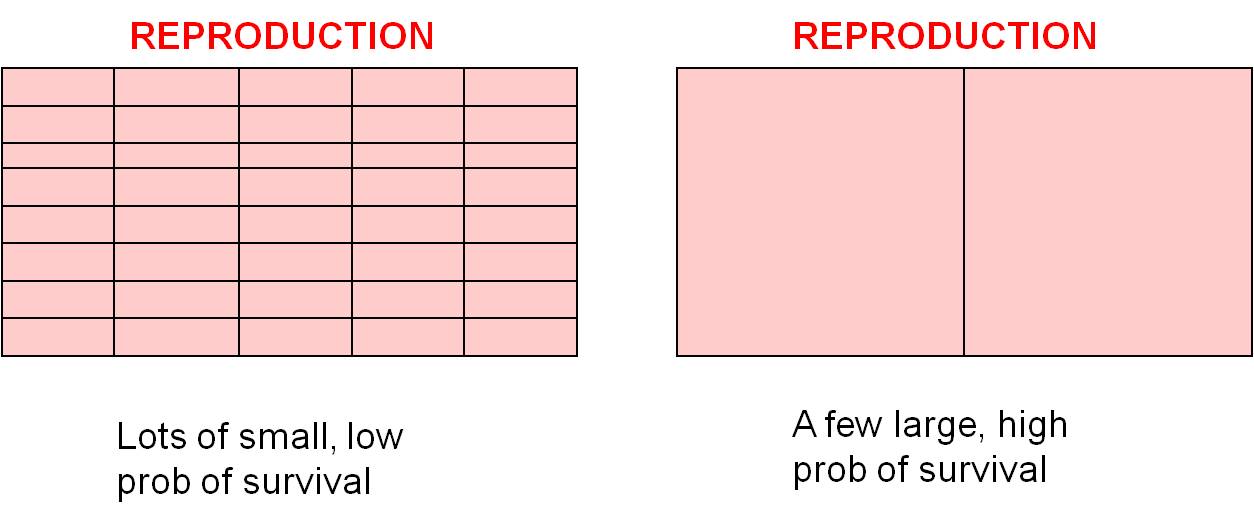Trade
Off #2: Offspring Number vs. Offspring Size/Survivorship: There are
also trade-offs within the reproduction budget (the pink section in the budgets,
above). For the same "energetic cost", you can make lots of little offspring
or a few big offspring. As we discussed above, small organisms have a lower
probability of survival than large organisms, so you can make lots of small
offspring that each have a small chance of surviving, or you can invest in fewer
offspring and increase their chance of survival (by making them larger or by
investments in parent care). Small organisms, like insects, can't really make
a big offspring, so they exploit the other strategy and make lots of small offspring.
Large organisms can really take advantage of this 'choice', and selection will
favor different strategies in different large organisms. Some organisms
like mammals and birds tend to produce a few large offspring and invest significant
amounts of energy in parental care, further increasing the probability that
the offspring survive. Large vertebrates without parental care, like many
reptiles, produce more numerous smaller offspring and 'play the lottery' - increasing
the chance that one offspring survives by producing more offspring.
b. Contradictory environmental pressures:
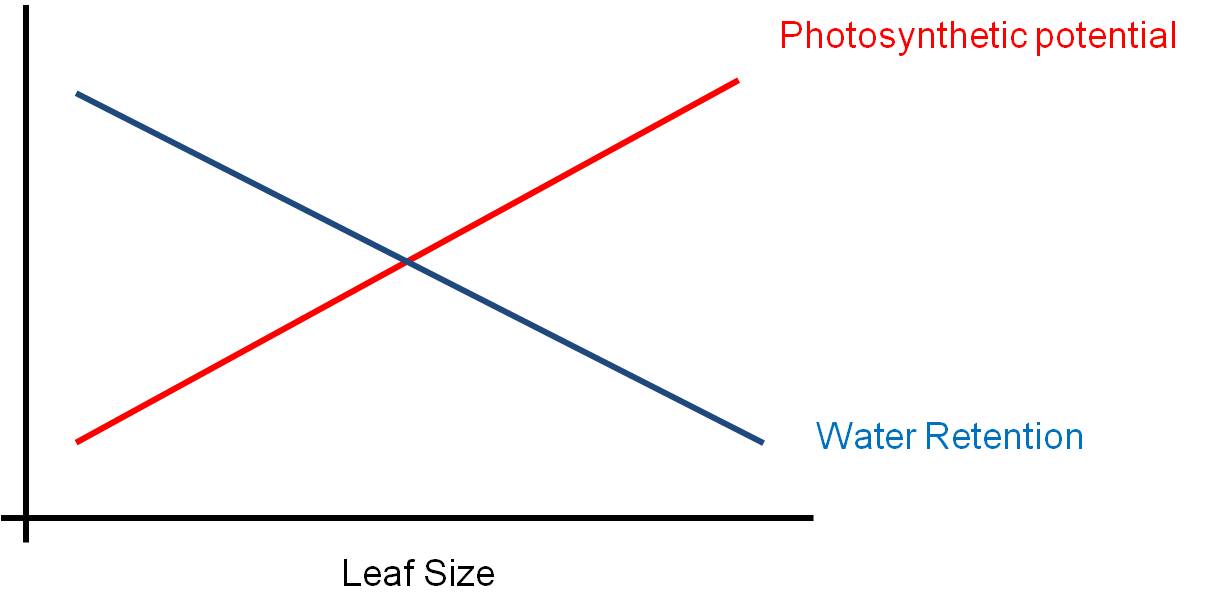
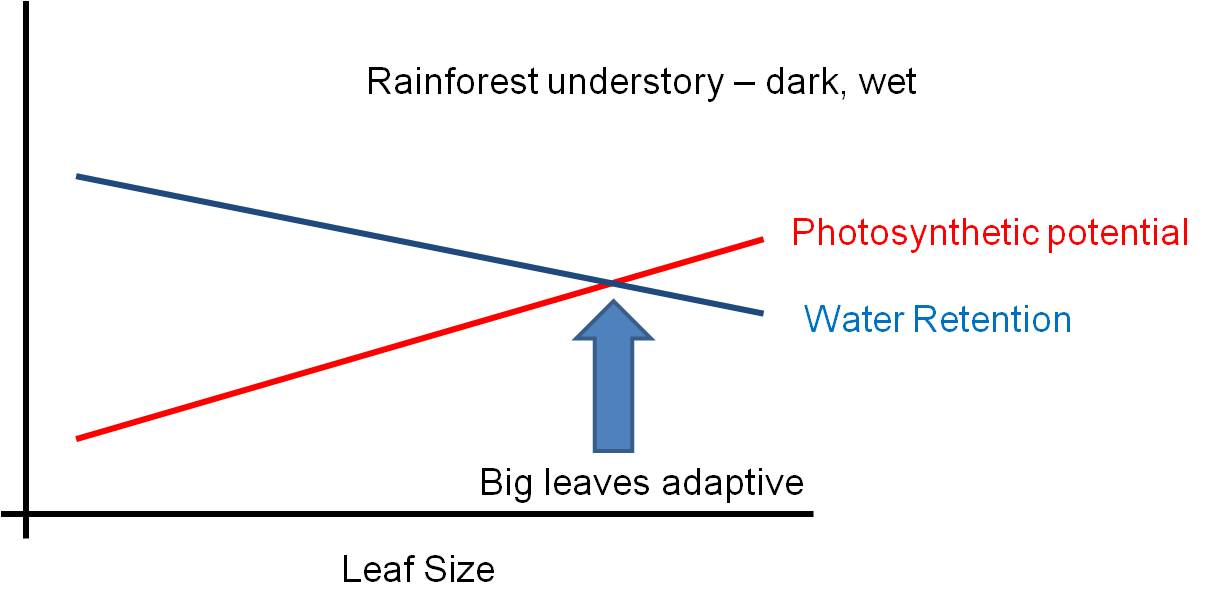
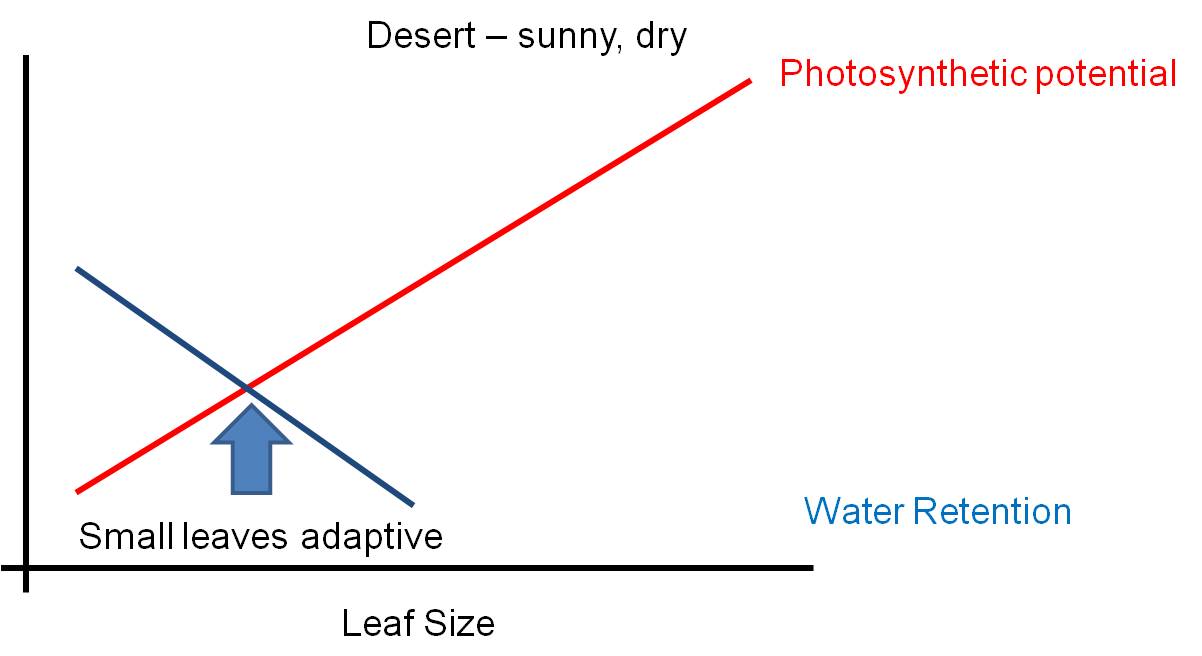
The environment is a complex place - there are pressures that might favor some structures, and other pressures acting AT THE SAME TIME that might select against that trait. Selection can not maximize BOTH responses at the same time - so the solution will be a suboptimal compromise. Consider leaf size. Big leaves are GOOD for light absorption - they represent a larger solar panel that intercepts more light. But big leaves are BAD for water loss - with a large SA/V ratio, water is lost rapidly from a large thin leaf. So, the size of a leaf will be a compromise solution to these two pressures... a solution that maximizes neither function. The 'adaptive compromise' size depends on the relative strengths of the contradictory pressures. If the risk of water loss is low (like in a rainforest), then the leaf can be large. If the risk of water loss is high (like in the desert)and there is strong sun, then the selective pressure to reduce leaf size is strong and leaves will be small or non-existent (cacti).
For these reasons, 'perfect' adaptations are impossible; there are biological costs to any adaptation (in terms of 'opportunity costs' - NOT being able to do something else as well),and in terms of the complex nature of the environment where selective pressures can be contradictory.
 |
 |
 |
3. Modeling Selection:
a.
Calculating relative fitness:
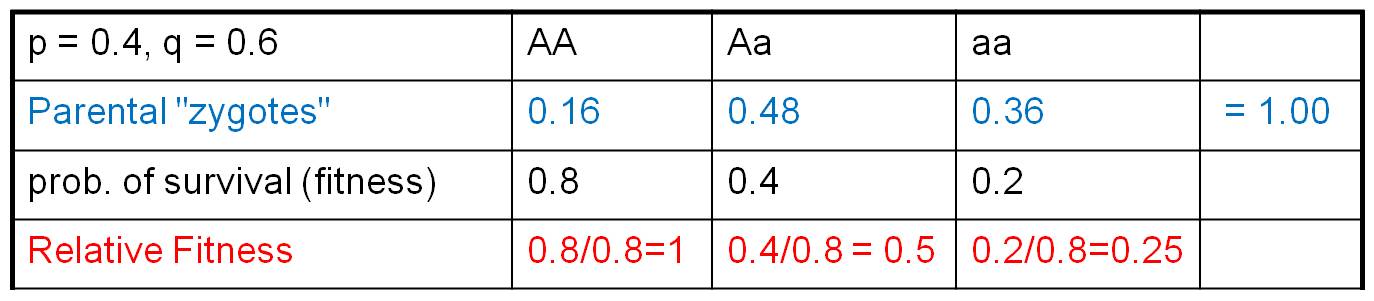
Consider the population to the right. Suppose a population has JUST mated, creating parental zygotes in the genotypic frequencies shown. Let's suppose that these zygotes, as a consequence of their genotypes at this locus, have different probabilities of surviving to reproductive age. But, to make things simple, lets assume that the other two components of fitness are equal across the genotypes. So, differences in FITNESS are only influenced by differences in the probability of survival to reproductive age. Suppose those survival probabilities are 0.8, 0.4, and 0.2, respectively (as shown).
These probabilities of survival are 'absolute' fitness values. Curiously, absolute fitness values are not very informative. What is important is relative reproductive success - reproductive success relative to the other genotypes in the population. Think about it this way... suppose I tell you that "I am thinking of an AA genotype that produces 1000 offspring a year. Do you think the f(A) will increase or decrease in the population as a consequence of selection?" Well, you might think, "wow, 1000 offspring is ALOT! Surely the f(A) will increase!" But this would be an incorrect assumption. You need to know what type of organism I am talking about, and how reproductively successful the other genotypes are in the population. If this organism is a salmon, then 1000 offspring might be more than other genotypes in the population. But if this organism is a clam, then the other genotypes might be producing 10's of thousands of offspring - and the F(A) will decline. So, the key to selection is differential reproductive success, which means reproductive success relative to other organisms in the population. We represent this as RELATIVE FITNESS, and calculate it by dividing all fitness values by the LARGEST (ie., most FIT) value. This means that one genotype will necessarily have a RELATIVE FITNESS = 1. In our case, above, we divide each fitness value by the greatest value (0.8), so the relative fitness values are "1, 0.5, and 0.25" for AA, Aa, and aa genotypes, respectively.
b.
Modeling Selection:
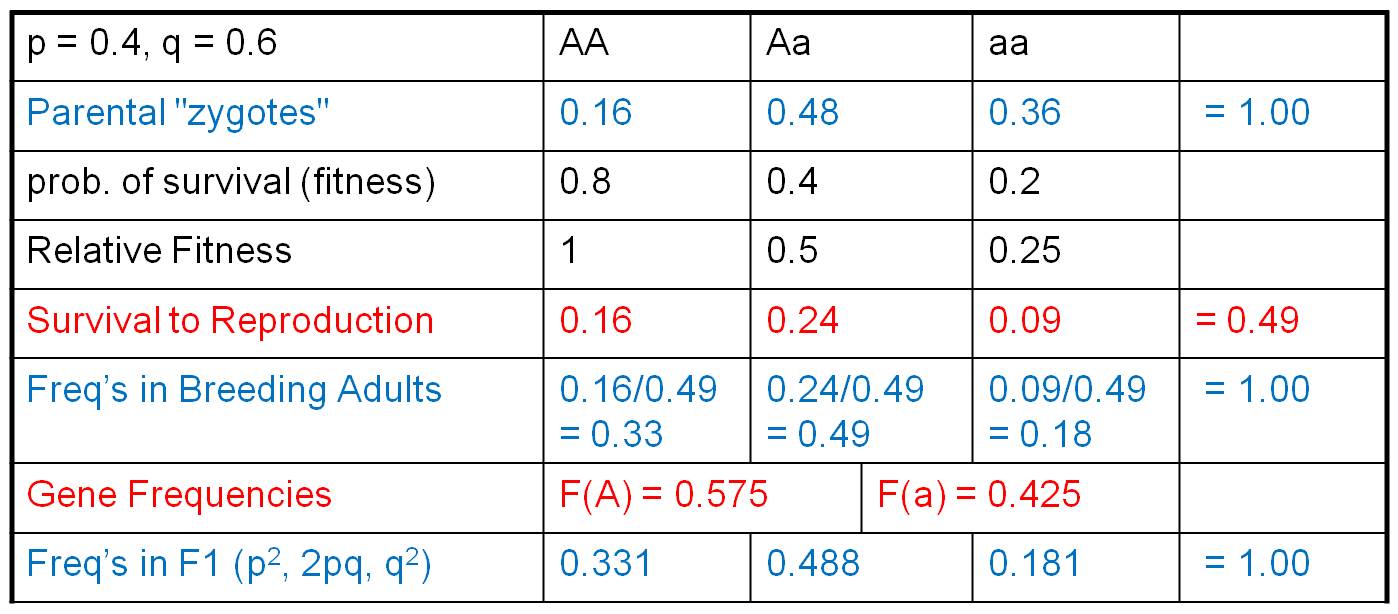
Now let's see what effect this selection
(in terms of differential survival) has on the genetic structure of the population.
First, we multiply the initial genotypic array by the relative fitness values.
For us, this describes the relative proportion of zygotes surviving to reproductive
age. So, these are the same organisms - we don't have a new generation yet -
it is just that the original zygotes have grown up and survived to adulthood
at different rates. Since many organisms have died, these genotypic values no
longer sum to 1. They sum to 0.49. If we want to know what the genotypic frequencies
are in the population of surviving reproductive adults (and WE DO), then we
must know what part of this new total is represented by each genotype. We calculate
that by dividing each genotypic value by the total (0.49), producing the genotypic
frequencies in this breeding population. These, of course, now sum to 1. From
here, we are home free. we calculate the gene frequencies in this reproductive
gene pool as:
F(A) = f(AA) = f(Aa)/2 = 0.575, and
f(a) = f(aa) + f(Aa)/2 = 0.425.
These sum to 1 so we've done the math correctly. Now, to produce the F1 zygotes,
assume that all other conditions of HWE are met (we are only modelling the direct
effect of selection, alone), so we assume that the organisms mate randomly and
calculate the genotypic frequencies of zygotes in the F1 using the terms: p2,
2pq, and q2.
So, as the result of differential survival to reproductive age, A's and a's have not been transferred at the same rate. The intial frequency of these genes was f(A) = 0.4 and f(a) = 0.6. After one generation of differential reproductive success, the gene frequencies have changed dramatically; now the 'A' gene is more abundant.
4.
Types of Selection
Selection can act in a number of ways in a population. Selection can favor one extreme phenotype over other phenotypes. This is called "directional selection", and the mean phenotype in the population (and the frequency of genes that influence the phenotype) should move in one direction. There is also "stabilizing" selection, in which an intermediate phenotype is most adaptive (think of sickle cell anemia in the tropics, where the heterozygote had the greatest probability of survival and reproduction). There is also "disruptive selection", in which both extremes are more adaptive than the intermediate type. Although this may seem unusual, it can be common for populations that exploit a complex environment. One phenotype might work well in one microenvironment within that habitat, the other extreme phenotype might work well in another specific microenvironment, but the intermediate might not do well in either. Consider the pollination example we used earlier for positive assortative mating. If there is a population of four o'clocks living in a habitat with no diurnal pollinating insects, then the pink flowers are selected against (don't reproduce) while the red and white flowers are selected for.
Darwin recognized another very important type of selection that he called "sexual selection". He realized that some traits in certain species might DECREASE the probability of survival, and yet might be adaptive. So, the long tail feathers in a peacock reduce survival: they hinder the bird from flying, and they also make it really easy for predators to see it. However, Darwin realized that these traits could still be adaptive if the cost of decreased survival was outweighed by the gain in reproduction while alive. So, Darwin appreciated the trade-offs that were possible in fitness components. Competition among members of one sex for access to the other is a type of sexual selection, too. When male bighorned sheep bash their heads and fight, they reduce their probability of survival because they can get hurt. However, winners increase their reproductive success so much that it compensates for the cost of battle in terms of relative fitness.
The period from 1900-1940 was a very exciting and dynamic period in biology. With the rediscovery of Mendel's principles, it seemed that biology had become a truly mathematical, predictive science. The ability to predict patterns of heredity, and the transmission of 'mutant' genes through generations, had a profound impact on evolutionary biology, too. Many geneticists came to view mutation as the primary agent of evolutionary change - not just as a primary source of variation. They viewed Darwin's ideas of probabilistic Natural Selection as too weak to be responsible for the changes seen in the fossil record. However, supporters of Darwin like Ernst Mayr argued that random mutation could not explain the non-random adaptations that were so obvious and pervasive in the natural world. The models of Hardy and Weinberg, in the hands of new population geneticists like Theodosius Dobzhansky and Sewall Wright, were pivotal in resolving this dilemma. The resolution came in the Modern Synthetic Theory of Evolution, developed by these scientists and others in the 1930's and 1940's. As a consequence of the models you have just seen, biologists realized that mutations were too rare to explain the changes seen in natural populations over time. Rather, although mutation and recombination were important as a source of new genetic variation, natural selection and genetic drift were the primary agents that caused the genetic structure of population to change over time. And so, as of 1940, our model of evolution looked like this:
Study Questions:
1. Why is relative fitness more important than fitness?
2. Consider the following population of zygotes:
AA Aa aa
Genotypic Frequency 0.3 0.3 0.4
Prob. of survival
0.4
0.2
0.1
a. What are the initial gene frequencies?
b. Is the population in HWE?
c. What are the relative fitness values?
d. What are the genotypic frequencies in the population of reproductive adults?
e. What are the gene frequencies in the population of reproductive adults?
f. If there is random mating, what will be the genotypic frequencies in the next generation?
g. What agent of evolutionary change is at work?
3. Outline the modern synthetic theory of evolution.
4. List the three components of fitness, and explain two trade-offs that necessarily occur because of limited energy budgets.
5. Why can selection perfect an organism? Describe in terms of contradictory selective pressures, and provide an example.